Answer:
(-6, 4)
Explanation:
We are given the following two equations and we are to solve them:
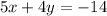 --- (1)
--- (1)
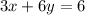 --- (2)
--- (2)
Using the substitution method:
From equation (2):
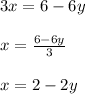
Substituting this value of x in equation (1) to get:
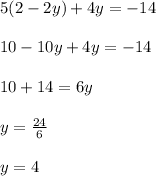
Putting this value of y in equation (2) to find the value of x:
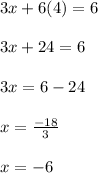
Therefore, (-6, 4) is the solution to the given system of equations.