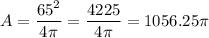Question

The circumference of a circle is 65?. In terms of pi, what is the area of the circle?

Answer 1

1056.25π square units

Explanation:

A few formulas an definitions which will help us:

(1)
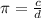`\pi=(c)/(d)`, where c is the circumference of a circle and d is its diameter

(2)
`A=\pi r^2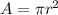`, where A is the area of a circle with radius r. To put it in terms of d, remember that a circle's diameter is simply twice its radius, or mathematically, (3)
`d=2r \rightarrow r=(d)/(2)`.

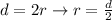We can rearrange equation (1) to put d in terms of π and c, giving us (4)
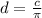`d = (c)/(\pi)`, and we can make a few substitutions in (2) using (3) and (4) to get use the area in terms of the circumference and π:

`A=\pi r^2\\=\pi\left((d)/(2)\right)^2\\=\pi\left((d^2)/(4)\right)\\=\pi\left(((c/\pi)^2)/(4)\right)\\=\pi\left((c^2/\pi^2)/(4)\right)\\=\pi\left((c^2)/(4\pi^2)\right)\\\\=(\pi c^2)/(4\pi^2)\\ =(c^2)/(4\pi)`

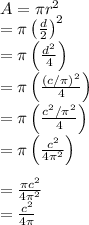
We can now substitute c for our circumference, 65, to get our answer in terms of π:

`A=(65^2)/(4\pi)=(4225)/(4\pi)=1056.25\pi`

Answer 2

Area = 2112.5 / pi

Explanation:

They are asking you not to use 3.14 for pi. Just leave it as a symbol.

C = 2*pi*r

C = 65

65 = 2*pi*r

65/(2*pi) = r

The area of a circle is 2*pi * r^2

Area = 2 * pi * (65/2pi)^2

Area = 2 * pi * 65^2/(4*pi^2) Cancel out one of the pi-s in the denominator

Area = 2 * 65^2 / (4 * Pi) Expand the numerator

Area = 8450/(4*pi) Divide by 4

Area = 2112.5 / pi