Question

4400 dollars is placed in an account with an annual interest rate of 8.25%.how much will be in the account after 22 years, to the nearest cent?

Answer 1

To find out how much will be in the account after 22 years with an annual interest rate of 8.25%, the compound interest formula
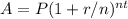`A = P(1 + r/n)^(nt)` is used, resulting in approximately $21739.63.

Step-by-step explanation:

To calculate how much money will be in the account after 22 years with an annual interest rate of 8.25%, we will use the compound interest formula:

`A = P(1 + r/n)^(nt)`

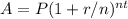
Where:

A is the amount of money accumulated after n years, including interest.

P is the principal amount (the initial amount of money).

r is the annual interest rate (decimal).

n is the number of times that interest is compounded per year.

t is the time the money is invested for, in years.

In this case:

P = $4400

r = 8.25% or 0.0825

n = 1 (since it's compounded annually)

t = 22 years

We plug these values into the compound interest formula:

`A = 4400(1 + 0.0825/1)^(1*22)`

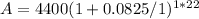
After calculating:

`A = 4400(1 + 0.0825)^(22)`

`A = 4400(1.0825)^(22)`

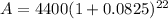
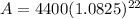
A comes out to approximately $21739.63, which is the total amount in the account to the nearest cent after 22 years.