ANSWER
all real numbers less than or equal to 4.
Step-by-step explanation
The given function is
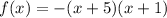
Expand
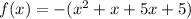
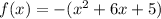
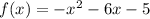
a=-1,b=-6,c=-5
The x-value of the vertex is given by
a=-1,b=-6,c=-5
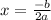
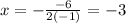
The y-value of the vertex is

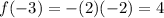
Since a=-1, the function opens downwards.
The highest value is the y-value of the vertex which is 4.
Therefore the range is all real numbers less than or equal to 4.
The correct choice is A.