Question

What are the horizontal and vertical components of a

300 N force that is applied along a rope at 60° to the

horizontal and used to drag an object across a yard?


help! can u explain the solve? idk why my answer is always reverse, like the answer for horizontal, not for vertical. the answer for vertical, not for horizontal. so, i am confusing. pls help!

if u got the answer, thanks​

Answer 1

In the figure attached we can see in a better way this situation. If we are told the rope is at 60 degress to the horizontal, we can place this rope in the origin of the X-Y coordinate system.

If we observe in detail, we have a right triangle where the opposite side
`OS` to the angle
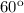`60\º` is the vertical component
`F_(y)` and the adjacent side
`AS` is the horizontal component
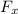`F_(x)` . So, we can use trigonometric functions to find the force
`F` (the hypotenuse
`h`) applied to the rope.

For
`F_(x)`:

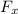We can use the trigonometric function cosine, which is defined as:

`cos(60\º)=(AS)/(h)`

This means
`F_(x)=Fcos(60\º)`

`F_(x)=300Ncos(60\º)`

`F_(x)=150N`

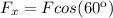
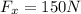
For
`F_(y)`:

We can use the trigonometric function sine, which is defined as:

`sin(60\º)=(OS)/(h)`

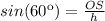
This means
`F_(y)=Fsin(60\º)`

`F_(y)=300Nsin(60\º)`

`F_(y)=259.8N`

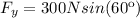
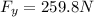
Finally the horizontal and vertical components are 150 N and 259.8 N