Question

What are the values of the variables in the triangle below? if the answer is not an integer, leave it in simplest radical form. the diagram is not drawn to scale

Answer 1

`x = 69,y = 23 √(3)`

EXPLANATION

Recall and use the mnemonics SOH CAH TOA.

We use the cosine ratio to find x.

`\cos(30 \degree) = (adjacent)/(hypotenuse)`

`\cos(30 \degree) = (x)/(46 √(3) )`

`( √(3) )/(2) = (x)/(46 √(3) )`

Cross multiply,

`2x = 46 √(3) * √(3)`

`2x = 46(3)`

`x = 23(3)`

`x = 69`

We use the sine ratio, to find y.

`\sin(30 \degree) = (opposite)/(hypotenuse)`

`\sin(30 \degree) = (y)/(46 √(3) )`

`(1)/(2) = (y)/(46 √(3) )`

Solve for y.

`(1)/(2) * 46 √(3) = y`

`23 √(3) = y`

Therefore,

`x = 69,y = 23 √(3)`

Answer 2

x = 69 and y =
`23√(3)`

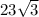
Explanation:

Firstly the hypotenuse is the side opposite the 90 degree angle. So hypotenuse is
`46√(3)`

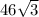
Since the angle given is 30 degree, with respect to this angle, the side length y is opposite and the side length x is adjacent.

Now, we can use trigonometric ratios to solve for x and y. Sine is defined as
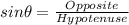`sin\theta=(Opposite)/(Hypotenuse)` and Cos is defined as
`Cos\theta=(Adjacent)/(Hypotenuse)`

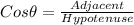
Hence, we can write:

`Sin(30)=(y)/(46√(3) )\\y=46√(3)*Sin30 \\y=46√(3)*(1)/(2)\\y=23√(3)`

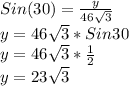
Also, we can figure out:

`Cos(30)=(x)/(46√(3) )\\Cos(30)*46√(3)=x\\ x=(√(3) )/(2)*46√(3) \\x=(46*3)/(2)\\x=69`

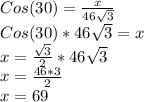
2nd answer choice is right.