Question

When the temperature of 2.35 m^3 of a liquid is increased by 48.5 degrees Celsius, it expands by 0.0920 m^3. What is its coefficient of volume expansion? (The answer is ________ •10^-4 C^-1. Just fill in the blank.)

Answer 1

8.1 is correct

Step-by-step explanation:

Just clarifying. 100% credit to the person above

Answer 2

`8.1\cdot 10^(-4) C^(-1)`

Step-by-step explanation:

The volumetric expansion of the liquid is given by

`\Delta V=\alpha V_0 \Delta T`

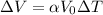
where

`\alpha` is the coefficient of volume expansion

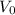`V_0` is the initial volume

`\Delta T` is the change in temperature

For the liquid in this problem,

`V_0 = 2.35 m^3\\\Delta T=48.5^(\circ)C\\\Delta V=0.0920 m^3`

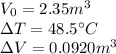
So we can solve the equation to find
`\alpha`:

`\alpha=(\Delta V)/(V_0 \Delta T)=((0.0920 m^3))/((2.35 m^3)(48.5^(\circ)C))=8.1\cdot 10^(-4) C^(-1)`