Question

Which function is the inverse of f(x) =b^x

Answer 1

The correct answer is C.

Explanation:

To find this, we need to note that in order to reverse the function of raising the b to the x power, we need to take the log base b of the function. Since the answer C is the only one containing that, it must be the correct answer,

Answer 2

The inverse of the function f(x) =
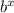`b^x` is the logarithmic function with base b, denoted as
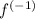`f^{(-1)`(x) = log_b(x).

The inverse of the function f(x) =
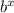`b^x` is the logarithmic function with base b, denoted as
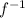`f^{-1`(x) = log_b(x). This means that for any value y,
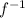`f^{-1`(y) is the value x such that f(x) = y. In other words, if f(x) =
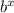`b^x`, then
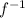`f^{-1`(y) = x when
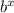`b^x`= y.

To see why this is true, consider the following equation:

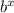`b^x` = y

Taking the logarithm of both sides with base b, we get:

log_b(
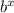`b^x`) = log_b(y)

Since log_b(
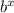`b^x`) = x, we have:

x = log_b(y)

Therefore,
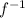`f^{-1`(y) = log_b(y).