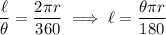The length of an arc subtended by a central angle is proportional to the circle's circumference. If the given central angle has measure
 and the circle has radius
and the circle has radius
 , then the arc has length
, then the arc has length
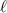 such that
such that
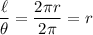
where
 is given in radians. Then
is given in radians. Then
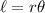
If
 is given in degrees, then
is given in degrees, then