Question

What are the solutions to the equation 3x^2 + 15x = 18. Show your work.

Answer 1

Answer: x1=1

x2=-6

Explanation:

3x^2+15x-18=0

D^2=225-4*3*(-18)/6=411

D=21

x1=(-15+21)/6=1

x2=(-15-21)/6=-6

Answer 2

Assignment:
`\bold{Solve \ Equation: \ 3x^2+15x=18}`

<><><><><><><>

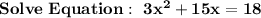
Answer:
`\boxed{\bold{x=1,\:x=-6}}`

<><><><><><><>

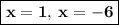
Explanation:
`\downarrow\downarrow\downarrow`

<><><><><><><>

[ Step One ] Subtract 18 From Both Sides

`\bold{3x^2+15x-18=18-18}`

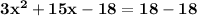
[ Step Two ] Simplify

`\bold{3x^2+15x-18=0}`

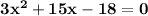
[ Step Three ] Solve With Quadratic Formula

Note:
`\bold{For\:a\:quadratic\:equation\:of\:the\:form\: ax^2+bx+c=0}`

`\bold{the \ solutions \ are \ x_(1,\:2)=(-b\pm √(b^2-4ac))/(2a)}`

`\bold{a=3,\:b=15,\:c=-18:\quad x_(1,\:2)=(-15\pm √(15^2-4\cdot \:3\left(-18\right)))/(2\cdot \:3)}`

<><><><><><><>

`\bold{(-15+√(15^2-4\cdot \:3\left(-18\right)))/(2\cdot \:3): \ 1}`

`\bold{(-15-√(15^2-4\cdot \:3\left(-18\right)))/(2\cdot \:3): \ -6}`

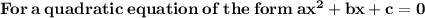
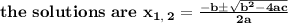
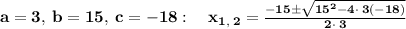
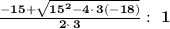
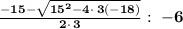
[ Step Four ] Combine Solutions

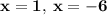
`\bold{x=1,\:x=-6}`

<><><><><><><>

`\bold{\rightarrow Mordancy \leftarrow}`