Answer: The value of x is 57.8.
Step-by-step explanation: We are given to find the value of x to the nearest tenth.
We can see from the figure that
the triangle is a right-angled triangle with one of the legs measuring 11 units and the hypotenuse is 13 units in length.
For the acute angle measuring x°, the perpendicular measures 11 units.
So, we get
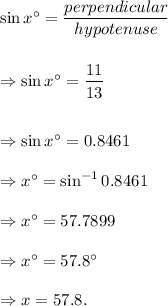
Thus, the value of x is 57.8.