Answer:
Explanation:
The center of a circle can be found using the equation
 and is (h,k) from it. Notice h and k are the opposite value as in the equation.
and is (h,k) from it. Notice h and k are the opposite value as in the equation.
First write the equation in this form.

Complete the square with each variable to find what numbers should go in place of the question marks.
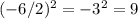
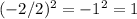
Add 1 and 9 to both sides of the equation.
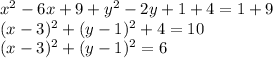
So the center is (3,1) and the radius is √6.