Question

A man sits with his back against the back of a chair, and he pushes a block of mass m = 6 kg straight forward on a table in front of him, with a constant force F = 34 N, moving the block a distance d = 0.7 m. The block starts from rest and slides on a low-friction surface. (Due to the nature of this problem, do not use rounded intermediate values in your calculations—including answers submitted in WebAssign.) (a) How much work does the man do on the block? J (b) What is the final kinetic energy K of the block? J (c) What is the final speed v of the block? m/s (d) How much time Δt does this process take? s (e) Consider the system of the man plus the block: how much work does the chair do on the man? J (f) What is the internal energy change of the man? J Now suppose that the man is sitting on a train that is moving in a straight line with speed V = 16 m/s, and you are standing on the ground as the train goes by, moving to your right. From your perspective (that is, in your reference frame), answer the following questions. (g) What is the initial speed vi of the block? m/s (h) What is the final speed vf of the block? m/s (i) What is the initial kinetic energy Ki of the block? J (j) What is the final kinetic energy Kf of the block? J (k) What is the change in kinetic energy ΔK = Kf − Ki? J How does this compare with the change in kinetic energy in the man's reference frame? This is greater than the same quantity in the man's reference frame. This is less than the same quantity in the man's reference frame. This is equal to the same quantity in the man's reference frame. (l) How far does the block move (Δx)? m (m) How much work does the man do on the block? J How does this compare with the work done by the man in his reference frame, and with ΔK in your reference frame? This is greater than the same quantity in the man's reference frame and it matches the ΔK in this frame. This is less than the same quantity in the man's reference frame and it matches the ΔK in this frame. This is equal to the same quantity in the man's reference frame and it doesn't match the ΔK in this frame. (n) How far does the chair move? m (o) Consider the system of the man plus the block: how much work does the chair do on the man? J How does this compare with the work done by the chair in the man's reference frame? This is greater than the same quantity in the man's reference frame. This is less than the same quantity in the man's reference frame. This is equal to the same quantity in the man's reference frame. (p) What is the internal energy change of the man? J How does this compare with the internal energy change in his reference frame? This is greater than the same quantity in the man's reference frame. This is less than the same quantity in the man's reference frame. This is equal to the same quantity in the man's reference frame

Answer 1

(a) 23.8 J

The work done by the man on the block is equal to the product between the force applied by the man (F=34 N) and the displacement of the box (d=0.7 m):

`W=Fd=(34 N)(0.7 m)=23.8 J`

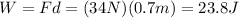
(b) 23.8 J

According to the work-energy theorem, the kinetic energy gained by the block is equal to the work done on it:

`\Delta K= K_f - K_i = W`

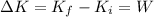
but since the block started from rest, its initial kinetic energy was zero:
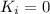`K_i = 0`, so the final kinetic energy is simply equal to the work done:

`K_i = W = 23.8 J`

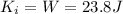
(c) 2.8 m/s

The final kinetic energy of the block is given by:

`K=(1)/(2)mv^2`

where m = 6 kg is the block's mass and v is the final speed. Solving the equation for v, we find

`v=\sqrt{(2K)/(m)}=\sqrt{(2(23.8 J))/(6 kg)}=2.8 m/s`

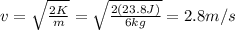
(d) 0.49 s

The impulse is defined as the product between the force applied (F) and the time taken (
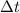`\Delta t`), and it is equal to the change in momentum of the block:

`I=F\Delta t = \Delta p = m \Delta v`

where F = 34 N, m = 6 kg, and
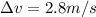`\Delta v=2.8 m/s` is the change in velocity of the block (the initial velocity was zero). Solving for
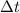`\Delta t`, we find

`\Delta t = (m \Delta v)/(F)=((6 kg)(2.8 m/s))/(34 N)=0.49 s`

(e) 0

The man does not move, therefore the work done by the chair on the man is zero, because the displacement is zero: d = 0, so in the formula W = Fd, the result is zero.

(f) -23.8 J

According to the law of conservation of energy, energy cannot be created nor destroyed. Therefore, when the man does work on the box, he must spend some energy, and the amount of this energy must be equal to the work done on the block. Since the man has lost energy, we must also put a negative sign, so the change in internal energy of the man is -23.8 J.

(g) 16 m/s

The initial velocity of the block in the frame of reference of the train is zero:
`v=0`

Since the reference frame (the train) is moving to the right at speed

`v_r = 16 m/s`

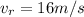
then the initial velocity of the block must be

`v'=v+v_r =0+16 m/s=16 m/s`

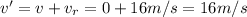
(h) 18.8 m/s

The final velocity of the block in the frame of reference of the train is 2.8 m/s, calculated at step c)
`v=0`

Since the reference frame (the train) is moving to the right at speed

`v_r = 16 m/s`

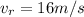
then the initial velocity of the block must be

`v'=v+v_r =2.8 m/s+16 m/s=18.8 m/s`

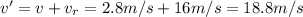
(i) 768 J

The initial kinetic energy of the block is given by:

`K_i = (1)/(2)mv_i^2`

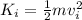
where m = 6 kg and
`v_i = 16 m/s`. Substituting numbers, we find

`K_i = (1)/(2)(6 kg)(16 m/s)^2=768 J`

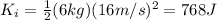
(j) 1060.3 J

The final kinetic energy of the block is given by:

`K_f = (1)/(2)mv_f^2`

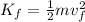
where m = 6 kg and
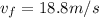`v_f = 18.8 m/s`. Substituting numbers, we find

`K_f = (1)/(2)(6 kg)(18.8 m/s)^2=1060.3 J`

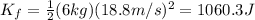
(k) 292.3 J

The change in kinetic energy is given by the difference between the kinetic energy calculated at point (j) and at point (i):

`\Delta K=K_f - K_i=1060.3 J-768 J=292.3 J`

and this is greater than the same quantity in the man's reference frame.

(l) 8.52 m

The total time of the motion is
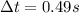`\Delta t=0.49 s` (calculated at step d). The initial velocity of the block is
`v_i = 16 m/s` and its acceleration is given by

`a=(F)/(m)=(34 N)/(6 kg)=5.67 m/s^2`

So we can calculate the displacement of the block by using

`\Delta x = v_i \Delta t + (1)/(2)a(\Delta t)^2=(16 m/s)(0.49 s)+(1)/(2)(5.67 m/s^2)(0.49 s)^2=8.52 m`

(m) 289.7 J

The work done by the man on the block in this reference frame is equal to the product between the force on the block and the displacement:

`W=Fd=(34 N)(8.52 m)=289.7 J`

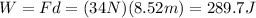
The change in kinetic energy of the block in this reference frame is

`\Delta K=K_f - K_i=1060.3 J-768 J=292.3 J`

This is greater than the same quantity in the man's reference frame and it matches the ΔK in this frame (the small difference is just due to the different rounding)

(n) 7.84 m

The chair just moved by uniform motion together with the train, so its displacement is given by

`d=v\Delta t=(16 m/s)(0.49 s)=7.84 m`

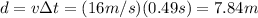
(o) 0

The chair and the man are moving together, so the chair is doing no work on the man, therefore the work done by the chair is still zero.

This is equal to the same quantity in the man's reference frame.

(p) -289.7 J

Due to the law of conservation of energy, the energy lost by the man must be equal to the work he has done. Since the work done by the man was 289.7 J, then the change in internal energy of the man must be -289.7 J.

This is greater than the same quantity in the man's reference frame.