Question

Is x^2 + 1/x = 4 a quadratic equations​

Answer 1

`\large\boxed{\pink{ \leadsto The \ given \ equation \ is \ not \ a \ Quadratic \ equation . }}`

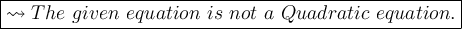
Explanation:

Given equation to us is ,

`\green{\implies x^2+(1)/(x)=4 }`

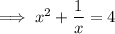
So , a equation is said to be a quadratic equation if the highest degree of the variable is 2 . On simplifying the Equation ,

`\implies x^2 +(1)/(x)=4`

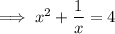
Taking x as LCM ,

`\implies (x^2.x + 1 )/(x)= 4`

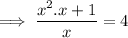
Transposing x to RHS .

`\implies x^3 + 1 = 4x`

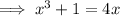
Putting all terms in LHS

`\boxed{\bf \implies x^3 - 4x - 1 = 0 }`

Since here the highest degree of the variable is 3 not 2 . So its a cubic equation and not a quadratic equation .

Hence the given equation is not a quadratic equation .