Answer: 8320 cubes.
Explanation:
1. Calculate the volume of the rectangular prism:
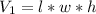
Where l is the lenght, w is the width and h is the height.
Substitute values.
(You can convert 3 1/4 to decimal by dividing the numerator by the denominator of the fraction and add this to the whole part: 3+0.25=3.25)
Then:
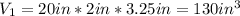
2. Calculate the volume of a cube:
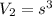
Where s is the lenght of any side.
Then:

3. To calculate the number of cubes that are needed to fill the rectangular prism (which you can call n) , you must divide the volume of the prism by the volume of a cube. Then:
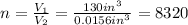 cubes
cubes