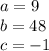Question

You pick a card at random from an ordinary deck of 52 cards. If the card is an ace, you get 9 points; if not, you lose 1 point.



Write the equation for the expected value.

E(V) =  452 (a) +  b52 (c)

a =  b =  c = 

​

Answer 1

Answer: for question 2, 3 and 4 on that page the answers are 2. -3/13 points: no you should not play the game . 3. E(X)=0 4. 12

Explanation:

Answer 2

`a = 9\\b = 48\\c = -1`

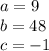
Explanation:

We know that:

In a deck of 52 cards there are 4 aces.

Therefore the probability of obtaining an ace is:

P (x) = 4/52

The probability of not getting an ace is:

P ('x) = 1-4 / 52

P ('x) = 48/52

In this problem the number of aces obtained when extracting cards from the deck is a discrete random variable.

For a discrete random variable V, the expected value is defined as:

`E(V) = VP(V)`

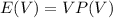
Where V is the value that the random variable can take and P (V) is the probability that it takes that value.

We have the following equation for the expected value:

`E(V) = (4)/(52)(a) + (b)/(52)(c)`

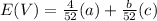
In this problem the variable V can take the value V = 9 if an ace of the deck is obtained, with probability of 4/52, and can take the value V = -1 if an ace of the deck is not obtained, with a probability of 48 / 52

Therefore, expected value for V, the number of points obtained in the game is:

`E(V) = (4)/(52)(9) + (48)/(52)(-1)`

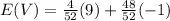
So:

`a = 9\\b = 48\\c = -1`