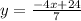Question

(20,-8); 7x - 4y = -5

Answer 1

The question is incomplete.

However, from the given parameters, a likely question could be to:

1. Write an equation in slope intercept form through (20,-8) and is parallel to 7x - 4y = -5

or

2. Write an equation in slope intercept form through (20,-8) and is perpendicular to 7x - 4y = -5

Answer:

See Explanation

Explanation:

First, we calculate the slope of
`7x - 4y = -5`

`7x - 4y = -5`

Subtract 7x from both sides

`7x-7x - 4y = -5-7x`

`- 4y = -5-7x`

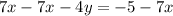
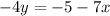
Make y the subject

`(- 4y)/(-4) = (-5-7x)/(-4)`

`y = (-5-7x)/(-4)`

`y = (5+7x)/(4)`

`y = (5)/(4)+(7)/(4)x`

`y = (7)/(4)x+(5)/(4)`

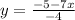
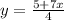
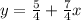
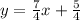
The general format of an equation is:

`y= mx + b`

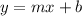
Where

`m = slope`

By comparison:

`m = (7)/(4)`

Solving (1): Parallel

Here, we assume that the line is parallel to the given equation.

And as such, it means that they have the same slope

So, we have:

`(x_1,y_1) = (20,-8)`

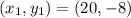
and

`m = (7)/(4)`

The equation is then calculated as:

`y - y_1 = m(x - x_1)`

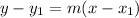
This gives:

`y - (-8) = (7)/(4)(x - 20)`

`y +8 = (7)/(4)(x - 20)`

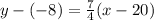
Open bracket

`y +8 = (7)/(4)x - (7)/(4)*20`

`y +8 = (7)/(4)x - 7*5`

`y +8 = (7)/(4)x - 35`

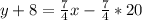
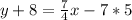
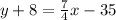
Make y the subject

`y = (7)/(4)x - 35-8`

`y = (7)/(4)x -43`

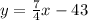
Solving (2): Perpendicular

Here, we assume that the line is perpendicular to the given equation.

And as such, it means that the following relationship exists between their slope:

`m_2 = -(1)/(m_1)`

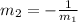
Where

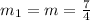`m_1 =m =(7)/(4)` -- as calculated above

Substitute 7/4 for m1 in
`m_2 = -(1)/(m_1)`

`m_2 = -(1)/(7/4)`

`m_2 = -(4)/(7)`

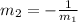
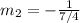
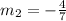
So, we have:

`(x_1,y_1) = (20,-8)`

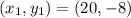
and

`m_2 = -(4)/(7)`

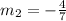
The equation is then calculated as:

`y - y_1 = m(x - x_1)`

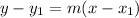
This gives:

`y - (-8) = -(4)/(7)(x - 20)`

`y +8 = -(4)/(7)(x - 20)`

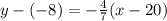
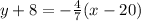
Open bracket

`y +8 = -(4)/(7)x + (4)/(7)*20`

`y +8 = -(4)/(7)x + (80)/(7)`

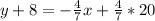
Make y the subject

`y = -(4)/(7)x + (80)/(7)-8`

`y = -(4)/(7)x + (80-56)/(7)`

`y = -(4)/(7)x + (24)/(7)`

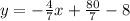
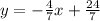
Take LCM

`y = (-4x + 24)/(7)`