Question

State whether it’s a function or not

Answer 1

Choice C is correct.

Explanation:

We have given a function :

f(x) = 1/x³

We have to find the inverse of function.

let y = f(x) we get,

y = 1/x³

Replacing x and y we get,

x = 1/y³

y³ = 1/x

Taking third roots on both sides we get,

y = 1/∛x

Replacing y to f⁻¹ (x) we get,

f⁻¹ (x)= 1/∛x is the inverse of f(x) = 1/x³.

So, As it is one to one and inverse of function exist so it is a function.

Answer 2

`\boxed{c. \ f^(-1)(x)=\frac{1}{\sqrt[3]{x}}}`

Explanation:

Let's find the inverse function of
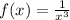`f(x)=(1)/(x^3)` to know what item we must choose as correct. So let's apply this steps:

a) Use the Horizontal Line Test to decide whether
`f` has an inverse function.

As shown in the graph below there is no any horizontal line that intersects the graph of
`f` at more than one point. Thus, the function is one-to-one and has an inverse function. Therefore, the inverse is a function..

b) Replace
`f(x)` by
`y` in the equation for
`f(x)`.

`y=(1)/(x^3)`

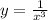
c) Interchange the roles of
`x` and
`y` and solve for
`y`

`x=(1)/(y^3) \\ \\ \therefore y^3=(1)/(x) \\ \\ \therefore y=\frac{1}{\sqrt[3]{x}}`

d) Replace
`y` by
`f^(-1)(x)` in the new equation.

`f^(-1)(x)=\frac{1}{\sqrt[3]{x}}`

So the correct option is:

`c. \ f^(-1)(x)=\frac{1}{\sqrt[3]{x}}`