Answer:
False
Explanation:
Let's find the inverse function of
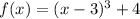 to know whether this function has an inverse function
to know whether this function has an inverse function
![f^(-1)(x)=-3+\sqrt[3]{x+4}](https://img.qammunity.org/2020/formulas/mathematics/high-school/l27hg0w402k55880rkt4pdzox898hf06v8.png) . So let's apply this steps:
. So let's apply this steps:
a) Use the Horizontal Line Test to decide whether
 has an inverse function.
has an inverse function.
Given that f(x) is a cubic function there is no any horizontal line that intersects the graph of
 at more than one point. Thus, the function is one-to-one and has an inverse function.
at more than one point. Thus, the function is one-to-one and has an inverse function.
b) Replace
 by
by
 in the equation for
in the equation for
 .
.
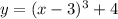
c) Interchange the roles of
 and
and
 and solve for
and solve for

![x=(y-3)^3+4 \\ \\ \therefore x-4=(y-3)^3 \\ \\ \therefore (y-3)^3=x-4 \\ \\ \therefore y-3=\sqrt[3]{x-4} \\ \\ \therefore y=\sqrt[3]{x-4}+3](https://img.qammunity.org/2020/formulas/mathematics/high-school/h7ui50maekyghr7t9hk3hjsdyk8y1nsujx.png)
d) Replace
 by
by
 in the new equation.
in the new equation.
![f^(-1)(x)=\sqrt[3]{x-4}+3](https://img.qammunity.org/2020/formulas/mathematics/high-school/m4auzvar1bu12mcym8gqnppwtsmmv8vdyw.png)
So this is in fact the inverse function and it isn't the same given function. Therefore, the statement is false