Question

Suppose f(x) = x^2. What is the graph of g(x) = 1/2f(x)?

Answer 1

Please see the attached image for your answer

Explanation:

If we use a graphing tool or calculator we can easily verify your expresions.

f(x) = x^2

g(x) = 1/(2f(x))

h(x) = (1/2)*f(x)

f(x) and h(x) differ only in their gain.

g(x) tends to zero as x tends to ±∞

Answer 2

Answer with explanation:

We are given a function f(x) in terms of variable x as:

`f(x)=x^2`

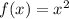
• We know that a transformation of a parent function f(x) of the type:

f(x) → a f(x)

is either a vertical stretch or a shrink depending on a.

If a>1 then the transformation is a vertical stretch and if a<1 then it is a vertical squeeze.

• Also, the transformation of the type:

f(x) → f(ax)

is a horizontal stretch if a<1 and a horizontal shrink if a>1.

• Here we have:

`g(x)=(1)/(2)f(x)`

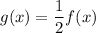
This means that the function g(x) is a vertical shrink of the parent function f(x) since a=1/2 <1

• Also, we can represent our function as:

`g(x)=((1)/(√(2))x)^2`

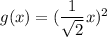
This means that:

`g(x)=f((1)/(√(2))x)`

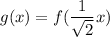
Here we have: a=1/√2 <1

This means that it is a horizontal stretch.