Question

A polynomial function has a root of –5 with multiplicity 3, a root of 1 with multiplicity 2, and a root of 3 with multiplicity 7. If the function has a negative leading coefficient and is of even degree, which statement about the graph is true? The graph of the function is positive on (-infinite, –5). The graph of the function is negative on (–5, 3). The graph of the function is positive on (-infinite, 1). The graph of the function is negative on (3, infinite).

Answer 1

The graph of the function is negative on (3, infinity).

Explanation:

I just got 100% on the quiz.

Answer 2

The root of -5 with multiplicity 3 implies that the polynomial is a multiple of

`(x+5)^3`

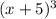
Similarly, the two other roots imply that the polynomial is a multiply of

`(x-1)^2(x-3)^7`

So, the minimal polynomial which satisfies your requests on the roots is

`(x+5)^3(x-1)^2(x-3)^7`

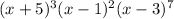
which would be a polynomial of degree 12. This polynomial would be:

• positive in
  `(-\infty, -5)`
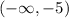
• negative in
  `(-5, -3)`
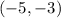
• positive in
  `(3, \infty)`

Since we want a negative leading term, the signs will be opposite: your polynomial is

• negative in
  `(-\infty, -5)`
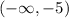
• positive in
  `(-5, -3)`
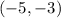
• negative in
  `(3, \infty)`

So, the only true statement is the last one.