Answer:
The ratio of lengths of the two mathematical pendulums is 9:4.
Step-by-step explanation:
It is given that,
The ratio of periods of two pendulums is 1.5
Let the lengths be L₁ and L₂.
The time period of a simple pendulum is given by :
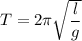
or
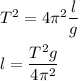
Where
l is length of the pendulum
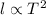
or
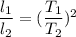 ....(1)
....(1)
ATQ,
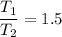
Put in equation (1)
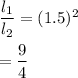
So, the ratio of lengths of the two mathematical pendulums is 9:4.