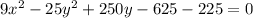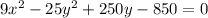Question

Q1: Identify the graph of the equation and write an equation of the translated or rotated graph in general form.

Answer 1

C. hyperbola;
`9x^2-25y^2-250y-850=0`

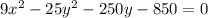
Explanation:

The given conic has equation:

`9x^2-25y^2=225`

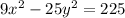
Divide through by 225.

`(9x^2)/(225)-(25y^2)/(225)=(225)/(225)`

`(x^2)/(25)-(y^2)/(9)=1`

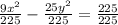
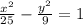
This is a hyperbola centered at the origin.

The hyperbola has been translated from the origin to (0,5).

The translated hyperbola will have equation;

`((x-0)^2)/(25)-((y-5)^2)/(9)=1`

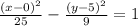
Multiply through by 225.

`9(x-0)^2-25(y-5)^2=225`

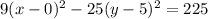
Expand

`9x^2-25(y^2-10y+25)=225`

`9x^2-25y^2+250y-625=225`

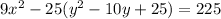
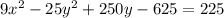
Rewrite in general form;

`9x^2-25y^2+250y-625-225=0`

`9x^2-25y^2+250y-850=0`