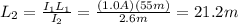Question

A large spool in an electrician's workshop has 55 m of insulation coated wire coiled around it. when the electrician connects a battery to the ends of the spooled wire, the resulting current is 1.0

a. some weeks later, after cutting off various lengths of wire for use in repairs, the electrician finds that the spooled wire carries a 2.6-a current when the same battery is connected to it. what is the length of wire remaining on the spool? m

Answer 1

21.2 m

Step-by-step explanation:

The current in the wire is given by Ohm's law:

`I=(V)/(R)`

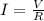
where V is the voltage of the battery and R is the resistance of the wire.

The resistance of the wire is directly proportional to the length of the wire, so we can write:

`R=kL`

where k is a constant and L is the length of the wire. Substituting into the first equation,

`I=(V)/(kL)`

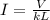
We can rewrite this equation also as

`(V)/(k)=IL`

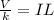
where the term on the left is a constant (because the voltage of the battery does not change). So, we can write:

`I_1 L_1 = I_2 L_2`

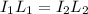
where:

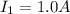`I_1 = 1.0 A` is the current in the first situation

`L_1 = 55 m` is the length of the wire in the first situation

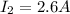`I_2 = 2.6 A` is the current in the second situation

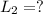`L_2=?` is the length of the wire in the second situation

Re-arranging the formula, we can find the value of L2:

`L_2 = (I_1 L_1)/(I_2)=((1.0 A)(55 m))/(2.6 m)=21.2m`