Question

4. A random variable X has a mean of 10 and a standard deviation of 3. If 2 is added to each value of X, what will the new mean and standard deviation be?

Answer 1

Adding 2 to each value of the random variable
`X` makes a new random variable
`X+2`. Its mean would be

`E[X+2]=E[X]+E[2]=E[X]+2`

since expectation is linear, and the expected value of a constant is that constant.
`E[X]` is the mean of
`X`, so the new mean would be

`E[X+2]=10+2=12`

The variance of a random variable
`X` is

`V[X]=E[X^2]-E[X]^2`

so the variance of
`X+2` would be

`V[X+2]=E[(X+2)^2]-E[X+2]^2`

We already know
`E[X+2]=12`, so simplifying above, we get

`V[X+2]=E[X^2+4X+4]-12^2`

`V[X+2]=E[X^2]+4E[X]+4-12^2`

`V[X+2]=(V[X]+E[X]^2)+4E[X]-140`

Standard deviation is the square root of variance, so
`V[X]=3^2=9`.

`\implies V[X+2]=(9+10^2)+4(10)-140=9`

so the standard deviation remains unchanged at 3.

NB: More generally, the variance of
`aX+b` for
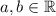`a,b\in\mathbb R` is

`V[aX+b]=a^2V[X]+b^2V[1]`

but the variance of a constant is 0. In this case,
`a=1`, so we're left with
`V[X+2]=V[X]`, as expected.