Answer:
Option d
Explanation:
We can solve this problem using the mathematical principles of permutations.
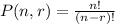
Where n is the number of things to choose and you choose r from them
To begin we must clarify that the English alphabet has 26 letters and there are 10 possible digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9.
Imagine then a plate that contains 6 characters. Three letters and three numbers.
{A, B, C, 1, 2, 3}
The first character can only be 1 of the following 5 letters I, D, A, H or O.
So:
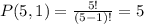
The next two characters can be any of the 26 letters of the alphabet.
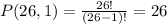
The last 3 characters can be any of the 10 numbers mentioned above.
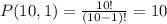
Therefore, the number of possible permutations is:
{5x26x26x10x10x10} = 3380000
To increase this number should allow the use of any of the 26 letters in the first character of the plate
{26x26x26x10x10x10} = 17576000
The correct answer is option d.
d. 5 x 26 x 26 x 10 x 10 x 10 x 10 – Allow for more first letters to be used.