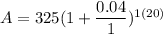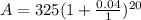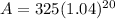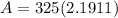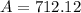Question

$325 is borrowed from a bank that charges 4% interest compounded annually. How much is owed after 1year ,3 years, 7 years & 20 years?

Answer 1

338 (1 Year)

365.58 (3 Years)

427.68 (7 Years)

712.12 (20 Years)

Explanation:

To find the compounded annual value, we use the formula:

`A = P(1 + r/n)^(nt)`

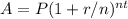
Let's first take each variable and list them:

P = 325

r = 4% or 0.04

t = 1, 3, 7, 20

n = 1

Let's take each number of years one at a time.

`A = P(1 + r/n)^(nt)`

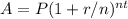
After 1 Year

`A = 325(1 + (0.04)/(1))^(1(1))`

`A = 325(1 + (0.04)/(1))^(1)`

`A = 325(1 + 0.04)^(1)`

`A = 325(1.04)^(1)`

`A = 325(1.04)`

`A = 338`

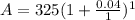
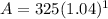
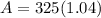
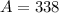
After 3 Years

`A = 325(1 + (0.04)/(1))^(1(3))`

`A = 325(1 + (0.04)/(1))^(3)`

`A = 325(1 + 0.04)^(3)`

`A = 325(1.04)^(3)`

`A = 325(1.1248)`

`A = 365.58`

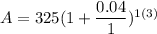
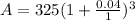
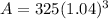
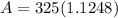
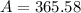
After 7 Years

`A = 325(1 + (0.04)/(1))^(1(7))`

`A = 325(1 + (0.04)/(1))^(7)`

`A = 325(1 + 0.04)^(7)`

`A = 325(1.04)^(7)`

`A = 325(1.3159)`

`A = 427.68`

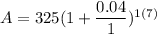
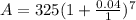
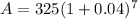
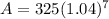
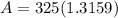
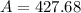
After 20 years

`A = 325(1 + (0.04)/(1))^(1(20))`

`A = 325(1 + (0.04)/(1))^(20)`

`A = 325(1 + 0.04)^(20)`

`A = 325(1.04)^(20)`

`A = 325(2.1911)`

`A = 712.12`