Answer:
y>-100 is the range.
Explanation:
Given is the function f(x) with domain the set of all real numbers
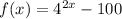
Let us substitute y =f(x)
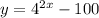
Let us solve x in terms of y to find the range
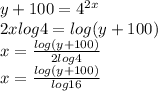
Since log is defined only for non negative positive numbers excluding 0, we have y+100>0
y>-100 is the range.