Question

An arrow is fired from a raised platform. Its height as a function of time is given by

h=-5t? + 10t + 40, where t is the time in seconds and h is the height, in metres.
a) Determine the height of the platform.
b) Find the length of time the arrow is in the air.
c) When will the arrow reach its maximum height? What is the maximum height of
the arrow?
d) When does the arrow have a height of exactly 45m above the ground?

Answer 1

(a) 40 m

(b) 4 seconds

(c) 1 second, 45 m

(d) 1 second

Explanation:

The given height as a function of height is

`h= -5t^2+10t+40`

where t is the time in seconds and h is the height, in meters.

(a) At the starting time, t=0, the arrow is at the platform.

So, putting t=0, to get the height of the platform

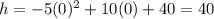`h= -5(0)^2+10(0)+40 = 40` m

(b) For determining the length of time the arrow is in the air, first t determining the value of t for which h=0, we have

`-5t^2+10t+40=0 \\\\-t^2+2t+8=0 \\\\-t^2+4t-2t+8=0 \\\\-t(t-4)-2(t-4)=0 \\\\(-t-2)(t-4)=0 \\\\`

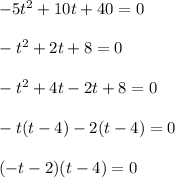
t=-2, t=4

Mathematically, the value of t for which the arrow is in the air is the time from -2 to 4 seconds.

But the arrow was at the platform at time t=0. So, neglecting the negative time, the time for which the arrow was in the air is the time from 0 to 4 seconds, i.e 4 seconds.

(c) Mathematically, the value of t for which the arrow is in the air is the time from t=-2 to t=4 seconds. So, in the middle of time, i.e at t=1 second the arrow will reach the maximum height.

Putting t=1 to get the maximum height, we have

`h=-5(1)^2+10(1)+40`

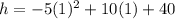
h=-5+10+40

h=45 m

Therefore, the maximum height is 45 m at t=1 second.

(d) Referinf option (c), at the time, t=1 second, the arrow has a height of exactly 45m above the ground