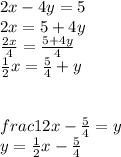Answer:
The equations are parallel! There is no solution.
Explanation:
Our equations are:
#1 x-2y=-8
#2 4x-8y=10
The first step is to see if it is possible to simplify either of the equations, which is possible for equation #2.
4x-8y=10 -> 2x-4y=5
#2 is now 2x-4y=5
Next, we must compare the slopes of the equations. If they are parallel, substitution or adding/subtraction methods for a system of equations will not work. Each equation will now be put into slope-intercept form; y=mx+b, where m equals the slope and b is the y intercept.
#1
x-2y=-8
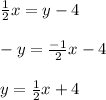
#2
2x-4y=5