Question

If f(x)=1/3x+7 and g(x)=-2x-5, find f(g(-7))

Please help.

Answer 1

`\boxed {\boxed {\sf f(g(-7))= 10}}`

Explanation:

We are asked to find f(g(-7)) given these 2 functions:

• 
  `f(x)=(1)/(3)x+7`

• 
  `g(x)= -2x-5`

We must work from the inside out, so first find g(-7).

1. g(-7)

The function for g is:

`g(x)= -2x-5`

Since we want to find g(-7), plug -7 in for x.

`g(-7)= -2(-7)-5`

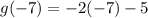
Solve according the PEMDAS: Parentheses, Exponents, Multiplication, Division, Addition, Subtraction.

Multiply -2 and -7.

`g(-7)=14-5`

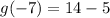
Subtract 5 from 14.

`g(-7)=9`

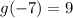
2. f(9)

Refer back to the original problem: f(g(-7))

Since we found that g(-7) is 9, we can substitute 9 in: f(9)

The function for f is:

`f(x)= (1)/(3) x+7`

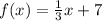
Plug 9 in for x.

`f(9)=(1)/(3)(9)+7`

Solve according to PEMDAS and multiply 1/3 and 9.

`f(9)=3+7`

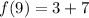
Add 3 and 7.

`f(9)=10`

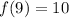
f(g(-7) is equal to 10

Answer 2

`f(g(-7))=10`

Explanation:

We have the two functions:

`\displaystyle f(x)=(1)/(3)x+7\text{ and } g(x)=-2x-5`

And we want to find:

`f(g(-7))`

So, we will first find g(-7). We know that:

`g(x)=-2x-5`

Then by substitution:

`g(-7)=-2(-7)-5`

Evaluate. Multiply:

`g(-7)=14-5=9`

Therefore, we can rewrite our expression as:

`f(g(-7))=f(9)`

Since we know that:

`\displaystyle f(x)=(1)/(3)x+7`

By substitution:

`\displaystyle f(9)=(1)/(3)(9)+7`

Evaluate:

`f(9)=3+7=10`

Therefore:

`f(g(-7))=10`