Question

Julio owns a four-sided lot that lies between two parallel streets. if his 90,000@ft2 lot has 500-ft frontage on one street and 300-ft frontage on the other, then how far apart are the streets?

Answer 1

Answer: The streets are 225 feet apart.

Explanation:

Since we have given that

Length of frontage on one street = 500 feet

Length of frontage on other street = 300 feet

Area of this figure = 90000 sq. feet

Let the distance between the street be 'h'.

Since it forms trapezium:

So, Area of trapezium would be

`90000=(1)/(2)* h* (a+b)\\\\90000=(1)/(2)* h* (500+300)\\\\90000* 2=800* h\\\\180000=800* h\\\\h=(180000)/(800)\\\\h=225\ ft`

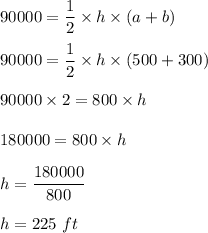
Hence, the streets are 225 feet apart.

Answer 2

225 ft

Explanation:

The area of a trapezoid can be found from the formula ...

A = (1/2)(b1 +b2)h

We can fill in the given information and solve for h. (All linear dimensions in ft; area in ft².)

90,000 = (1/2)(500 + 300)h

90,000/400 = h = 225

The distance between streets is 225 feet.