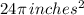Question

The sector of a circle with a 12-inch radius has a central angle measure of 60°.

What is the exact area of the sector in terms of ​ π ​?

Answer 1

Answer: Area of sector in term of π is 24 in.².

Explanation:

Since we have given that

Radius of the sector of a circle = 12 inch

Central angle = 60°

As we know the formula for "Area of sector":

`Area=(\theta)/(360^\circ)\pi r^2\\\\Area=(60)/(360)* \pi* 12* 12\\\\Area=24\pi\ inch^2`

Hence, Area of sector in term of π is 24 in.².

Answer 2

The exact area of the sector in terms of ​π is
`24\pi \thinspace inches^2`

Explanation:

Given the radius of circle 12-inch and the central angle measure of 60°.

We have to find the the exact area of the sector in terms of ​ π.

As the area of sector can be calculated by the formula

`A=(\theta)/(360^(\circ))*\pi r^2`

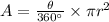
where
`\theta` is the central angle in degree and r is the radius of circle.

Now,
`A=(60)/(360^(\circ))*\pi (12)^2`

`=(1)/(6)*\pi (12)^2`

`=24\pi \thinspace inches^2`

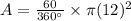
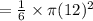
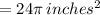
Hence, the exact area of the sector in terms of ​π is
`24\pi \thinspace inches^2`