Question

A sumo wrestling ring is circular and has a circumference of 4.6\pi \text{ meters}4.6? meters. What is the area AA of the sumo wrestling ring in square meters? Give your answer in terms of \pi?. A=A= \text{m}^2m 2

Answer 1

He is right

Explanation:

Answer 2

The area is equal to
`5.29 \pi\ m^(2)`

Explanation:

Step 1

Find the radius r

we know that

the circumference of a circle is equal to

`C=2\pi r`

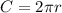
In this problem we have

`C=4.6 \pi\ m`

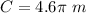
Substitute in the formula and solve for r

`4.6 \pi=2\pi r`

`r=4.6/2=2.3\ m`

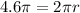
Step 2

Find the area

we know that

The area of a circle is equal to

`A=\pi r^(2)`

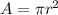
substitute the value of r

`A=\pi (2.3^(2))=5.29 \pi\ m^(2)`