Answer:
Options A and B are true
Explanation:
In this question it is given that population of a bacterial colony doubles every 10 minutes and the function that represents the number of bacteria in colony after x minutes is
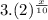
Now we check each option given
A. The expression
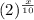 reveals the population of the bacterial colony increases by 100% every 10 minutes.
reveals the population of the bacterial colony increases by 100% every 10 minutes.
Let's check by putting x = 10
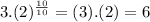
Here after 10 minutes initial population which was 3 got doubled to 6. So option A is true.
B. Expression
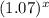 reveals the approximate rate of increase in the population of the bacterial colony per minute.
reveals the approximate rate of increase in the population of the bacterial colony per minute.
Let's solve the expression
![3.(2)^{(x)/(10)}=3.[(2)^{(1)/(10)}]^(x)=3.[1.07]^(x)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/x456sgcyppy09i23zvay79lcg7y3jnav5o.png)
Option B is true.
C. This option is not correct as we have already solved the expression in option B.
D. This option can't be correct because it is itself given in the question that
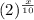 reveals that the bacterial colony increases by 100% in 10 minutes.
reveals that the bacterial colony increases by 100% in 10 minutes.
E. Let's check this option by putting x = 1 minute in
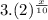
and in
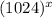 . If solutions of both the expressions are same then this option will be correct.
. If solutions of both the expressions are same then this option will be correct.
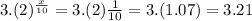
And
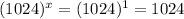
As both the solutions are different so Option E is incorrect.
F. As we have seen in option E both the expressions give different values for different values of x so Option F will be incorrect again.
Options A and B are true.