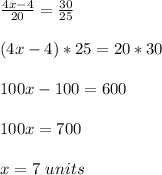Question

(a) Are these triangles similar and if so why? (what similarity postulate can you use to prove this)

(b) What is the value of x? Show ALL of your work.

Answer 1

Part a) The triangles KJL and NMP are similar by AAA similarity postulate

Part b) The value of x is
`7\ units`

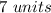
Explanation:

Part a)

we know that

The AAA similarity postulate states that If in two triangles the corresponding angles are equal, then the triangles are similar

In this problem

m<JKL=m<MNP

m<KJL=m<NMP

so

The third angle must be equal (remember that the sum of the interior angles of triangle must be equal to 180 degrees)

m<JLK=m<MPN

therefore

The triangles KJL and NMP are similar by AAA similarity postulate

Part b) What is the value of x?

we know that

if two figures are similar, then the ratio of its corresponding sides are equal

`(4x-4)/(20)=(30)/(25)\\ \\(4x-4)*25=20*30\\ \\100x-100=600\\\\ 100x=700\\ \\x=7\ units`