Question

Calculate m∠ADC. Round to the nearest tenth.

Answer 1

22.6 degrees to the nearest tenth.

Explanation:

Triangle ABC is a right angled triange so,

using the Pythagoras theorem for triangle ABC:

AC^2 = 5^2 + 12^2

AC^2 = 25 + 144 = 169

AC = 13.

Triangle ADC is also a right angled triangle, so:

sin < ADC = 13 / 33.8 = 0.3846

< ADC = 22.6 degrees.

Answer 2

Measure of ∠ADC = 22.62°

Explanation:

Firstly, we have right triangle ABC with AB = 12 cm and BC = 5 cm.

'Pythagoras Theorem' states that 'The sum of squares of the length of the sides in a right triangle is equal to the square of the length of the hypotenuse'.

That is,
`Hypotenuse^(2)=Perpendicular^(2)+Base^(2)`

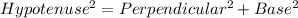
i.e.
`AC^(2)=12^(2)+5^(2)`

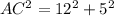
i.e.
`AC^(2)=144+25`

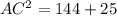
i.e.
`AC^(2)=169`

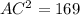
i.e.
`AC=\pm 13`

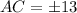
As, the length of the hypotenuse cannot be negative.

So, we get, AC = 13 cm.

Further, we have a right triangle ACD with perpendicular = AC = 13 cm and hypotenuse = AD = 33.8 cm.

As we know, 'In a right angled triangle, the angles and sides can be written in trigonometric forms'.

That is,
`\sin ADC=(perpendicular)/(hypotenuse)`

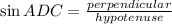
i.e.
`\sin ADC=(13)/(33.8)`

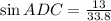
i.e.
`\sin ADC=0.3846`

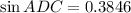
i.e.
`ADC=\arcsin 0.3846`

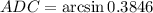
i.e.
`ADC=22.62`

Thus, the measure of ∠ADC = 22.62°