Answer:
b)Divergent
Explanation:
Given is a series
-5+25-125+....
We find that this is following a pattern such that each term is multiplied by -5 to get the next term
In other words, this series is a geometric series with common ratio r =-5 and I term a=-5
Since sum of n terms of geometric series =
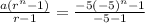
Since |r|=|-5|>1, we find that this sum tends to infinity or -infinity as n tends to infinity
In other words, the series diverges