Question

Use the ratio test to determine whether the series is convergent or divergent.

Answer 1

Option A is correct. since series is convergent.

Step-by-step explanation

nth term of the given series is given by

`a_(n) =(2n-1)/((2n-1)!)`

`a_(n+1) =(2n+1)/((2n+1)!)`
`\lim_(n \to \infty) (a_(n+1))/(a_(n) ) =((2n+1)/(2n+1!) )/((2n-1)/(2n-1!)  ) =((2n+1)X(2n-1)!)/((2n-1)X(2n+1)!)`

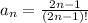
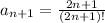
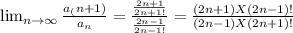
on simplifying it ,we get

`\lim_(n \to \infty)(1)/((2n-1)(2n))`

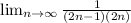
which gives zero at n = infinity

since value of the limit of the ratio is less than 1

given series is convergent [ by ratio test ]

Answer 2

a) Convergent by ratio test

Explanation:

Given is the series

`1+(3)/(1.2.3) +(5)/(1.2.3.4.5) +...`

General term =

`a_(n) =(2n-1)/((2n-1)!)`

To use ratio test

Let us write n+1 th term

=
`a_(n+1) =(2n+1)/((2n+1)!)`

Find ratio of n+1th term to nth term

`(a_(n+1) )/(a_(n) ) =(2n+1)/(2n-1) ((1)/(2n(2n+1) )`

We find that here numerator has degree as 1 and denominator as 3

SInce numerator has more powers, it approaches infinity faster than numerator thus making ratio tend to 0 as n becomes large

Since ratio tends to 0, we have this series is convergent.