Answer:
Option (b) is correct.
The sum of the first 70 terms of the sequence –6, –3, 0, 3, 6, ... is 6825.
Explanation:
Consider the given sequence, –6, –3, 0, 3, 6, ...
We have to find the sum of the first 70 terms of the sequence –6, –3, 0, 3, 6, ...
Here,
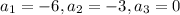 and so on.
and so on.
First find the difference between the terms,


Since, the difference between the terms is constant,Hence, the given sequence is an Arithmetic sequence.
For a given A.P. with n terms having a as first term and d be the common difference the sum
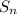 is given by,
is given by,
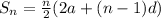
Here, a = -6 , n = 70 , d = 3 ,
Substitute , we get,

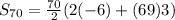

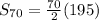
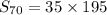
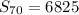
Thus, the sum of the first 70 terms of the sequence –6, –3, 0, 3, 6, ... is 6825.
Option (b) is correct.