Question

the gravitational force between two objects is 1600 and what will be the gravitational force between the objects if the distance between them doubles?

Answer 1

I believe this is what you have to do:

The force between a mass M and a point mass m is represented by

`F = G(Mm)/(r^(2) )`

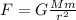
So lets compare it to the original force before it doubles, it would just be the exact formula so lets call that F₁

So F₁ = G(Mm/r^2)

Now the distance has doubled so lets account for this in F₂:

F₂ = G(Mm/(2r)^2)

Now square the 2 that gives you four and we can pull that out in front to give

F₂ =
`(1)/(4)` G(Mm/r^2)

Now we can replace G(Mm/r^2) with F₁ as that is the value of the force before alterations

now we see that:

F₂ =
`(1)/(4)` F₁

So the second force will be 0.25 (1/4) x 1600 or 400 N.