Answer:
C) domain: all real numbers greater than or equal to 0
range: all real numbers greater than or equal to 13,000
Explanation:
We have, the function for the cost of laying cable is given by,
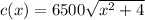 , where x is the length of the cable (in feet).
, where x is the length of the cable (in feet).
As, 'x' represents the length of the cable.
We have that, the value of x cannot be negative.
So, x ≥ 0.
Since, the domain of
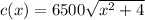 is the set of points where
is the set of points where
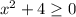 and we have that x ≥ 0.
and we have that x ≥ 0.
Thus, the domain is 'Set of all real numbers greater than or equal to 0'.
Now, we substitute x= 0 in
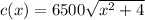 .
.
i.e.
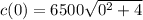
i.e.
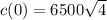
i.e.
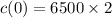
i.e. c(0) = 13000.
So, we get that the vertex point of the function is (0,13000).
Thus, the range is 'All real numbers greater than or equal to 13,000'.