Answer:
Option (b) is correct.
b)
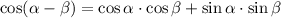
Explanation:
Given

To prove the above stated formula we have to choose one of the identity from the given options .
Since right side of above formula is
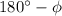 which is same as
which is same as
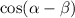 , we will use the identity
, we will use the identity
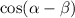
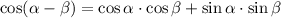
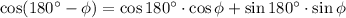
We know
 and
and

Substitute above, we get,

Thus, Option (b) is correct.