Question

Monthly water bills for a city have a mean of $108.43 and a standard deviation of $32.09. Find the probability that a randomly selected bill will have an amount greater than $155, which the city believes might indicate that someone is wasting water. Would a bill that size be considered unusual?

Answer 1

The probability that a randomly selected bill will have an amount greater than $155 is 0.07353.

No, a bill with $155 can not be considered unusual.

Explanation:

We have been given that monthly water bills for a city have a mean of $108.43 and a standard deviation of $32.09.

Let us find the z-score for the raw score 155, using z-score formula.

`z=(x-\mu)/(\sigma)`, where,

`z=\text{ z-score}`,

`x=\text{ Raw score}`,

`\mu=\text{Mean}`,

`\sigma=\text{Standard deviation}`.

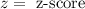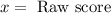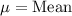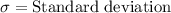Upon substituting our given values in above formula we will get,

`z=(155-108.43)/(32.09)`

`z=(46.57)/(32.09)`

`z=1.4512\approx 1.45`

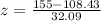
Let us find probability of z-score greater than 1.45.

`p(z>1.45)=1-P(z<1.45)`

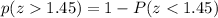
Using normal distribution table we will get,

`p(z>1.45)=1-0.92647`

`p(z>1.45)=0.07353`

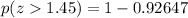
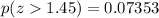
Therefore, the probability that a randomly selected bill will have an amount greater than $155 is 0.07353 or approximately 7.35%.

Since z-scores lower than -1.96 or higher than 1.96 are considered unusual. As the bill with size $155 has z-score of 1.45, therefore, a bill with size $155 can not be considered unusual.