Question

In trapezoid ABCD, AC is a diagonal and ∠ABC≅∠ACD. Find AC if the lengths of the bases BC and AD are 12m and 27m respectively.

Answer 1

Length of diagonal is 18 m

Explanation:

Given in trapezoid ABCD. AC is a diagonal and ∠ABC≅∠ACD. The lengths of the bases BC and AD are 12m and 27m. We have to find the length of AC.

Let the length of diagonal be x m

In ΔABC and ΔACD

∠ABC=∠ACD (∵Given)

∠ACB=∠CAD (∵Alternate angles)

By AA similarity theorem, ΔABC~ΔACD

∴ their corresponding sides are proportional

`(x)/(27)=(12)/(x)=(AB)/(CD)`

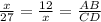
Comparing first two, we get

⇒
`(x)/(27)=(12)/(x)`

⇒
`x^2=27* 12`

⇒
`x=\sqrt324=18`

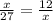
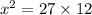
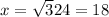
hence, the length of diagonal is 18 m