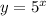Question

Match each function formula with the corresponding transformation of the parent function y = 5-^x Please help ASAP!!

1. y = 5x
Translated left 5 units
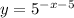
2. y = 5–x + 5
Translated right by 5 units
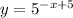
3. y = 5–x – 5
Translated up by 5 units
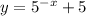
4. y = 5–x+ 5
Translated down by 5 units
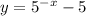
5. y = –5–x
Reflected across the x-axis
6. y = 5–x – 5
Reflected across the y-axis

Answer 1

1. Translated left 5 units
`y=5^(-x-5)`

2. Translated right by 5 units
`y=5^(-x+5)`

3. Translated up by 5 units
`y=5^(-x)+5`

4. Translated down by 5 units
`y=5^(-x)-5`

5. Reflected across the x-axis
`y=-5^(-x)`

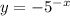
6. Reflected across the y-axis
`y=5^(x)`

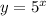
Explanation:

The given parent function is

`y=5^(-x)`

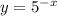
The transformation of this function is defined as

`y=5^(-(x+a))+b`

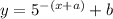
Where, a represents horizontal shift and b represents vertical shift.

If a>0, then graph shifts a units left and if a<0, then graph shifts a units right.

If b>0, then graph shifts b units up and if b<0, then graph shifts b units down.

1. Translated left 5 units

`y=5^(-x-5)`

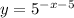
2. Translated right by 5 units

`y=5^(-x+5)`

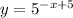
3. Translated up by 5 units

`y=5^(-x)+5`

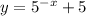
4. Translated down by 5 units

`y=5^(-x)-5`

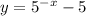
5. If graph reflected across the x-axis, then the graph passes through (x,-y).

`-y=5^(-x)`

`y=-5^(-x)`

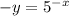
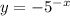
6. If graph reflected across the y-axis, then the graph passes through (-x,y).

`y=5^(-(-x))`

`y=5^(x)`

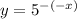
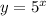
Therefore the required matching is

1. Translated left 5 units
`y=5^(-x-5)`

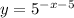
2. Translated right by 5 units
`y=5^(-x+5)`

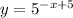
3. Translated up by 5 units
`y=5^(-x)+5`

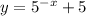
4. Translated down by 5 units
`y=5^(-x)-5`

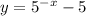
5. Reflected across the x-axis
`y=-5^(-x)`

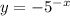
6. Reflected across the y-axis
`y=5^(x)`

Answer 2

We have the parent function given by,
`y=5^(-x)`

1. The function is translated 5 units to the left.

So, we get,
`y=5^(-x)` becomes
`y=5^(-(x+5))`

2. The function is translated 5 units to the right.

So, we get,
`y=5^(-x)` becomes
`y=5^(-(x-5))`

3. The function is translated 5 units upwards.

So, we get,
`y=5^(-x)` becomes
`y=5^(-x)+5`

4. The function is translated 5 units downwards.

So, we get,
`y=5^(-x)` becomes
`y=5^(-x)-5`

5. The function is reflected across x-axis.

So, we get,
`y=5^(-x)` becomes
`y=-5^(-x)`

6. The function is reflected across y-axis.

So, we get,
`y=5^(-x)` becomes
`y=5^(-x)`.