Question

A student submitted the following answer to the graphing systems problem:

Is that student correct? Why or why not? If not, what would the solution be?

Answer 1

Answer: No, she is not correct.

Explanation:

Since we have given that

`6x+y=36-----------(1)\\\\5x-y=8---------------(2)`

First we check the consistency of system of equations:

`(a_1)/(a_2)=(b_1)/(b_2)=(c_1)/(c_2)`

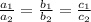
here ,
`a_1=6,b_1=1,c_1=36\\a_2=5,b_2=-1,c_2=8`

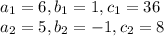
so, it becomes,

`(6)/(5)\\eq (1)/(-1)\\eq (36)/(8)`

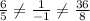
So, it is consistent and it is an intersecting lines.

So, it would have a unique solution.

From Eq(1), we have,

`6x+y=36\\\\y=36-6x`

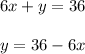
Put it in eq(2), we have

`5x-y=8\\\\5x-(36-6x)=8\\\\5x-36+6x=8\\\\11x-36=8\\\\11x=36+8\\\\11x=44\\\\x=(44)/(11)=4`

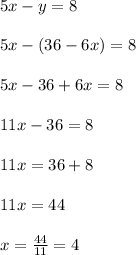
So, x=4 and

`y=36-6x=36-6* 4=36-24=12`

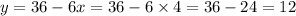
so, the solution point of this line will be (4,12).

No, she is not correct.