Answer:
The total percent decrease is
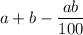
Explanation:
- We are given with a word problem
- We are asked to find the total percentage decrease
- We can do this in two steps
Step 1: Finding the two percentage decrease
Step 2: Finding their difference
Step 1 of 2
Let the decrease by a% be 100-a
Then b% decrease is
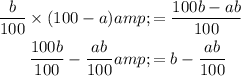
Step 2 of 2
The percentage difference between the two percentages is,
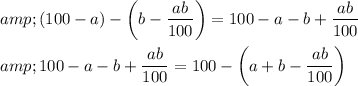
Since 100 is the total percent
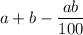 is the percentage decrease.
is the percentage decrease.