Answer:
The solution of the given set in interval form is
![$(-\infty,-4] \cup[12, \infty)$](https://img.qammunity.org/2023/formulas/mathematics/high-school/bwvrdtsuuo0i92h3bi8u1wu34q4r9h8zst.png) .
.
Explanation:
It is given in the question an inequality as
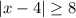 .
.
It is required to determine the solution of the inequality.
To determine the solution of the inequality, solve the inequality
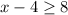 and,
and,
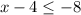
Step 1 of 2
Solve the inequality
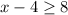
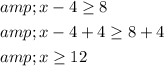
Solve the inequality
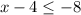 .
.

Step 2 of 2
The common solution from the above two solutions is x less than -4 and
 .
.
The solution set in terms of interval is
![$(-\infty,-4] \cup[12, \infty)$](https://img.qammunity.org/2023/formulas/mathematics/high-school/bwvrdtsuuo0i92h3bi8u1wu34q4r9h8zst.png) .
.