Question

Gavin goes for a run at a constant pace of 9 minutes per mile. Ten minutes later, Lars goes for a run, along the same route, at a constant pace of 7 minutes per mile. How many minutes does it take for Lars to reach Gavin?

Answer 1

Answer: The answer is 35 minutes.

Step-by-step explanation: Given that Gavin goes for a run at a constant pace of 9 minutes per mile and after 10 minutes, Lars started running along the same route, at a constant pace of 7 minutes per mile. We need to find the number of minutes Lars will take to reach Gavin.

In 9 minutes, distance run by Gavin = 1 mile.

So, in 1 minute, distance travelled by Gavin will be

`d_G=(1)/(9)=(7)/(63)~\textup{miles}.`

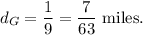
Similarly,

In 7 minutes, distance run by Lars = 1 mile.

So, in 1 minute, distance travelled by Lars will be

`d_L=(1)/(7)=(9)/(63)~\textup{miles}.`

Now, since Lars started after 10 minutes, so distance run by Gavin in those 10 minutes will be

`d_(G10)=(10)/(9)=(70)/(63)~\textup{miles}.`

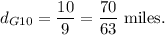
Now, difference between Lars and Gavin's rate of runnings is

`(9)/(63)-(7)/(63)=(2)/(63).`

Therefore, the time taken by Lars to reach Gavin is given by

`t=((70)/(63))/((2)/(63))=35.`

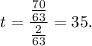
Thus, the required time is 365 minutes.